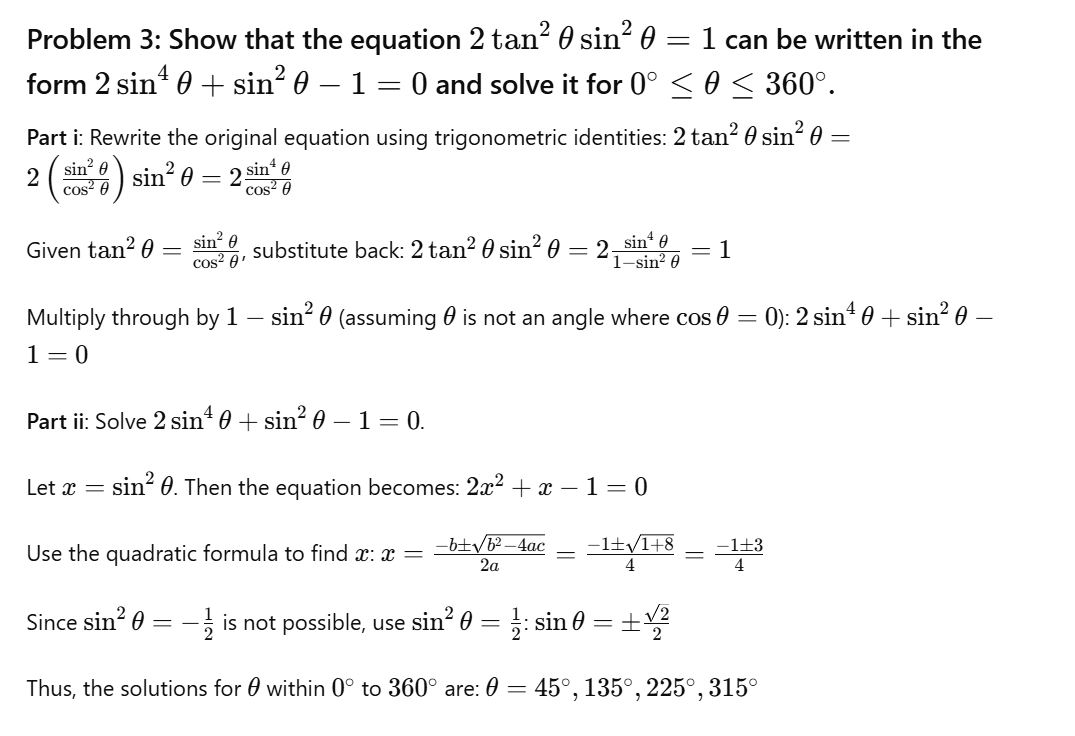
Trigonometric Identities and Equations
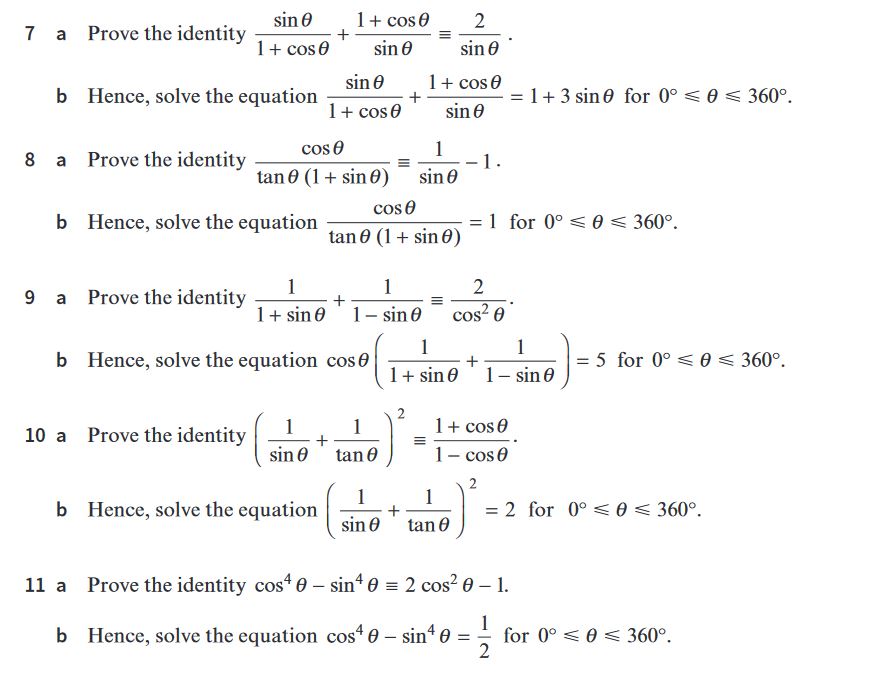
Question 7
(a) Prove the identity
\[ \frac{\sin \theta}{1 + \cos \theta} + \frac{1 + \cos \theta}{\sin \theta} = \frac{2}{\sin \theta} \]
Solution:
Step 1: Left-hand Side (LHS):
\[ \frac{\sin^2 \theta + (1 + \cos \theta)^2}{(1 + \cos \theta) \sin \theta} \]
Step 2: Expand \((1 + \cos \theta)^2\):
\[ \frac{\sin^2 \theta + 1 + 2 \cos \theta + \cos^2 \theta}{(1 + \cos \theta) \sin \theta} \]
Step 3: Combine \(\sin^2 \theta + \cos^2 \theta\) to get 1:
\[ \frac{1 + 2 \cos \theta + 1}{(1 + \cos \theta) \sin \theta} = \frac{2(1 + \cos \theta)}{(1 + \cos \theta) \sin \theta} \]
Step 4: Cancel \((1 + \cos \theta)\):
\[ \frac{2}{\sin \theta} \]
Conclusion: The identity is proven.
Question 8(b) Solve the equation
\[ \frac{\sin \theta}{1 + \cos \theta} + \frac{1 + \cos \theta}{\sin \theta} = 1 + 3 \sin \theta \]
Solution:
Step 1: Replace LHS with the proven identity:
\[ \frac{2}{\sin \theta} = 1 + 3 \sin \theta \]
Step 2: Multiply both sides by \(\sin \theta\):
\[ 2 = \sin \theta (1 + 3 \sin \theta) \]
Step 3: Expand the right side:
\[ 2 = \sin \theta + 3 \sin^2 \theta \]
Step 4: Rearrange into a quadratic equation:
\[ 3 \sin^2 \theta + \sin \theta - 2 = 0 \]
Step 5: Solve this quadratic equation using the quadratic formula:
\[ \sin \theta = \frac{-1 \pm \sqrt{1 + 4 \times 3 \times 2}}{2 \times 3} = \frac{-1 \pm \sqrt{25}}{6} = \frac{-1 \pm 5}{6} \]
Step 6: The solutions are:
\[ \sin \theta = \frac{4}{6} = \frac{2}{3} \quad \text{and} \quad \sin \theta = -1 \]
Step 7: Verify the range of \(\theta\) and find the corresponding values.
Question 8
(a) Prove the identity
\[ \frac{\cos \theta}{\tan \theta (1 + \sin \theta)} = \frac{1}{\sin \theta} - 1 \]
Solution:
Step 1: Left-hand Side (LHS):
\[ \frac{\cos \theta}{\frac{\sin \theta}{\cos \theta} (1 + \sin \theta)} = \frac{\cos^2 \theta}{\sin \theta (1 + \sin \theta)} \]
Step 2: Rewrite \(\cos^2 \theta\) using the Pythagorean identity:
\[ \frac{1 - \sin^2 \theta}{\sin \theta (1 + \sin \theta)} \]
Step 3: Simplify using partial fractions:
\[ \frac{1}{\sin \theta} - 1 \]
Conclusion: The identity is proven.
(b) Solve the equation
\[ \frac{\cos \theta}{\tan \theta (1 + \sin \theta)} = 1 \]
Solution:
Step 1: Use the proven identity:
\[ \frac{1}{\sin \theta} - 1 = 1 \] \[ \frac{1}{\sin \theta} = 2 \]
Step 2: Solve for \(\sin \theta\):
\[ \sin \theta = \frac{1}{2} \]
Step 3: Determine \(\theta\):
\[ \theta = 30^\circ, 150^\circ \]
Conclusion: The solutions are \(30^\circ\) and \(150^\circ\).
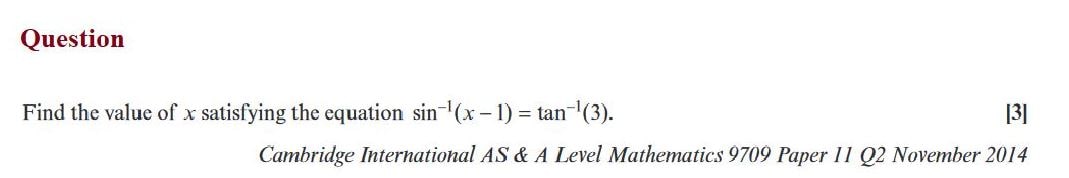
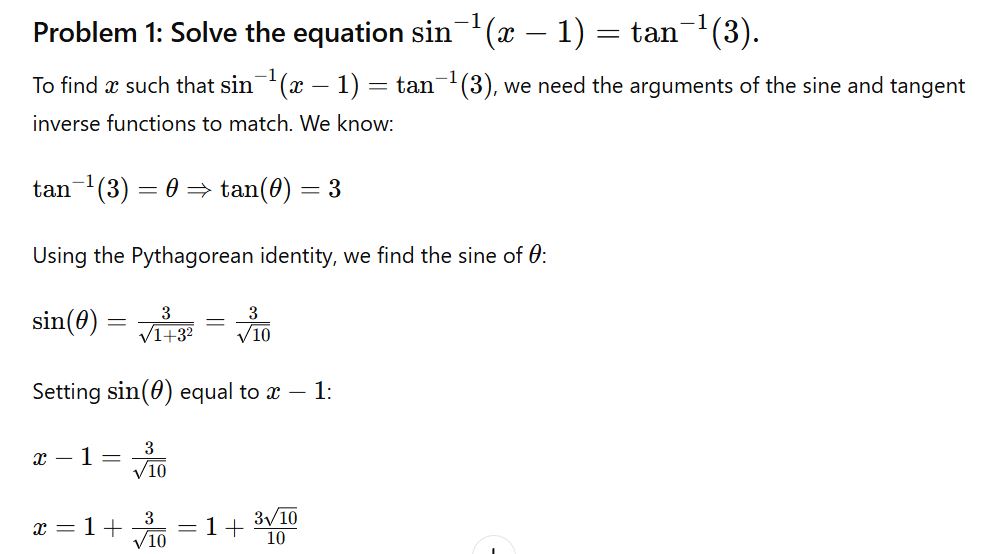
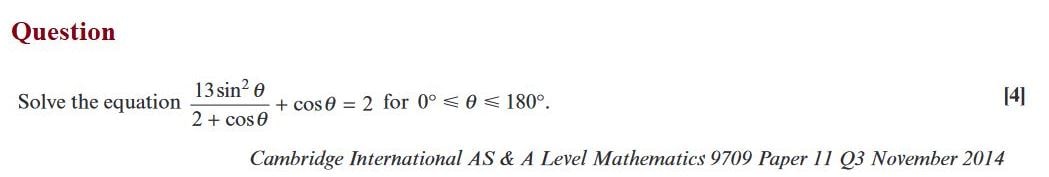


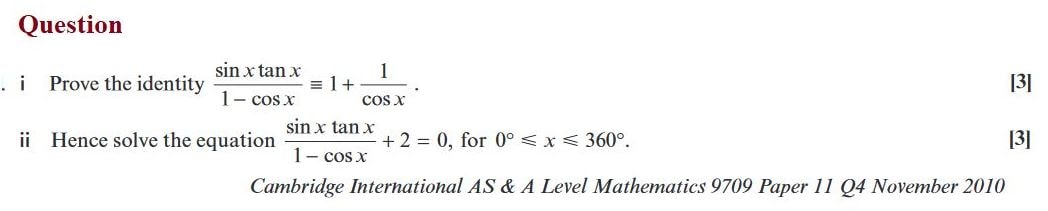

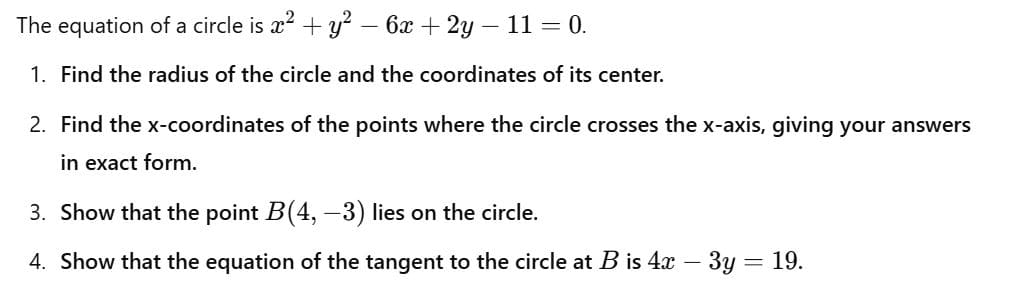
\[ \textbf{1a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 - 6x + 2y - 11 = 0 \] Completing the square: \[ (x - 3)^2 + (y + 1)^2 = 20 \] The center is \( (3, -1) \) and the radius is \( 2\sqrt{5} \). \[ \textbf{1b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 - 6x - 11 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{1c. Verifying Point B:} \] Substitute \( B(4, -3) \) into the original equation to confirm it satisfies the circle equation. \[ \textbf{1d. Tangent Equation at B:} \] Find the slope of \( OB \) and use it to find the slope of the tangent. Verify that the tangent equation is: \[ 4x - 3y = 19 \]
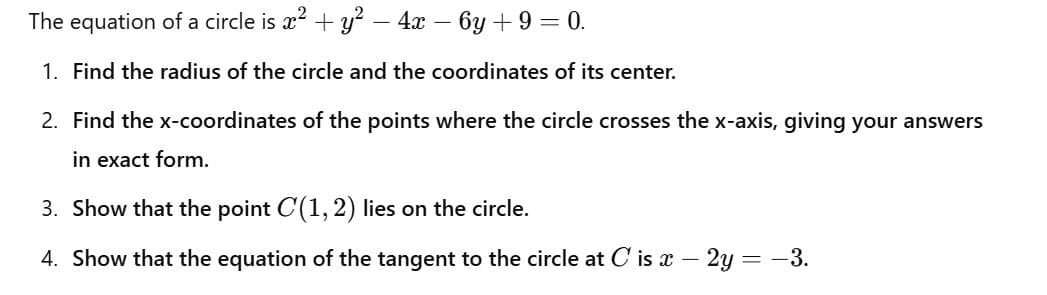
\[ \textbf{2a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 - 4x - 6y + 9 = 0 \] Completing the square: \[ (x - 2)^2 + (y - 3)^2 = 4 \] The center is \( (2, 3) \) and the radius is \( 2 \). \[ \textbf{2b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 - 4x + 9 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{2c. Verifying Point C lies on the circle:} \] Substitute \( C(1, 2) \) into the original equation to confirm it satisfies the circle equation.
Apparently we observe that the point C does not satisfy the circle equation.
This means the point C is not on the circle, but away from the circle.
The Basic IdeaThe idea to solve the problem would be to first find the equation of the line that connects the center with C(1,2)
For this, we will first find the Slope of the line, and then the equation of the line.
Our next step would be to find the point of intersection of the line with the circle.
To find this, all we need to do is to substitute the equation of the line into the equation of the circle and simplify.
We will get two points of,x, substitute these, and get the points for y, so now we have two coordinates, where the line intersects the circle.
It is these point of contact for which we will find the equation of the tangent.
Solution
Solution for Finding Tangent Line from External Point \(C(1, 2)\) to a Circle
The given circle's equation is in the form \((x-2)^2 + (y-3)^2 = 4\).
Step 1: Circle’s Center and Radius
The center of the circle \( (h, k) \) is \( (2, 3) \) and the radius \( r \) is 2.
Step 2: Line Equation from \( C \) to Circle's Center
The slope of the line connecting \( C(1, 2) \) and the center \( (2, 3) \) is \( m = 1 \). The line equation is \( y = x + 1 \).
Step 3: Intersection Points
Substitute \( y = x + 1 \) into the circle’s equation to find intersection points:
\((x-2)^2 + (x-2)^2 = 4 \Rightarrow 2(x-2)^2 = 4 \Rightarrow x - 2 = \pm \sqrt{2} \Rightarrow x = 2 \pm \sqrt{2} \)
Using \( x \) values, \( y = 3 \pm \sqrt{2} \)
Step 4: Equation of Tangents
Detailed Derivation of Tangent Lines to a Circle at Intersection Points
Using the points of intersection derived from the line y = x + 1 with the circle defined by \((x-2)^2 + (y-3)^2 = 4\), we derive the tangent lines.
Step 1: Calculate the Slope of the Radius
For the first intersection point (2 + \sqrt{2}, 3 + \sqrt{2}):
The radius connects the center of the circle (2, 3) to the point (2 + \sqrt{2}, 3 + \sqrt{2}). The slope of this radius (m_r) is:
\[ m_r = \frac{(3 + \sqrt{2}) - 3}{(2 + \sqrt{2}) - 2} = 1 \]
Step 2: Determine the Slope of the Tangent Line
The slope of the tangent (m_t) is the negative reciprocal of the radius's slope:
\[ m_t = -\frac{1}{m_r} = -1 \]
Step 3: Equation of the Tangent Line Using Point-Slope Form
Using the point-slope form of a line, and the point \(2 + \sqrt{2}, 3 + \sqrt{2}\):
\[ y - (3 + \sqrt{2}) = -1(x - (2 + \sqrt{2})) \]
\[ y - 3 - \sqrt{2} = -x + 2 + \sqrt{2} \]
\[ y = -x + 5 \]
This is the equation of the tangent line at the first intersection point. Following the same steps for the second point (2 - \sqrt{2}, 3 - \sqrt{2}) also leads to the equation:
\[ y = -x + 5 \]
Conclusion
Both intersection points \( (2 + \sqrt{2}, 3 + \sqrt{2}) \) and \( (2 - \sqrt{2}, 3 - \sqrt{2}) \) have the same tangent line equation: \( y = -x + 5 \). This symmetry illustrates a unique geometric relationship between the line, circle, and tangents.
\[ \textbf{3a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 + 2x - 4y - 4 = 0 \] Completing the square: \[ (x + 1)^2 + (y - 2)^2 = 9 \] The center is \( (-1, 2) \) and the radius is \( 3 \). \[ \textbf{3b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 + 2x - 4 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{3c. Verifying Point D:} \] Substitute \( D(-1, 1) \) into the original equation to confirm it satisfies the circle equation. \[ \textbf{3d. Tangent Equation at D:} \] Find the slope of \( OD \) and use it to find the slope of the tangent. Verify that the tangent equation is: \[ x + y = 0 \]\[ \textbf{Question 1} \] The equation of a circle is \( x^2 + y^2 - 10x + 8y - 16 = 0 \).
Find the equation of the tangent
if E(7,-2) is given as the point of contact.
Note: you are expected to perform a check to ascertain if indeed the point E, lies on the circle. \[ \textbf{1a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 - 10x + 8y - 16 = 0 \] Completing the square: \[ (x - 5)^2 + (y + 4)^2 = 25 \] The center is \( (5, -4) \) and the radius is \( 5 \). \[ \textbf{1b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 - 10x - 16 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{1c. Verifying Point E:} \] Substitute \( E(7, -2) \) into the original equation to confirm it satisfies the circle equation. \[ \textbf{1d. Tangent Equation at E:} \] Find the slope of \( OE \) and use it to find the slope of the tangent. Verify that the tangent equation is: \[ 7x + 2y = 42 \] \[ \textbf{Question 2} \] The equation of a circle is \( x^2 + y^2 + 4x - 12y + 4 = 0 \). \[ \textbf{2a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 + 4x - 12y + 4 = 0 \] Completing the square: \[ (x + 2)^2 + (y - 6)^2 = 40 \] The center is \( (-2, 6) \) and the radius is \( 2\sqrt{10} \). \[ \textbf{2b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 + 4x - 32 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{2c. Verifying Point F:} \] Substitute \( F(-1, 5) \) into the original equation to confirm it satisfies the circle equation. \[ \textbf{2d. Tangent Equation at F:} \] Find the slope of \( OF \) and use it to find the slope of the tangent. Verify that the tangent equation is: \[ x + y = 4 \] \[ \textbf{Question 3} \] The equation of a circle is \( x^2 + y^2 - 12x - 16y + 36 = 0 \). \[ \textbf{3a. Finding Radius and Center:} \] Given: \[ x^2 + y^2 - 12x - 16y + 36 = 0 \] Completing the square: \[ (x - 6)^2 + (y - 8)^2 = 64 \] The center is \( (6, 8) \) and the radius is \( 8 \). \[ \textbf{3b. X-Intercepts of the Circle:} \] Substitute \( y = 0 \): \[ x^2 - 12x + 20 = 0 \] Use the quadratic formula to find \( x \). \[ \textbf{3c. Verifying Point G:} \] Substitute \( G(8, -4) \) into the original equation to confirm it satisfies the circle equation. \[ \textbf{3d. Tangent Equation at G:} \] Find the slope of \( OG \) and use it to find the slope of the tangent. Verify that the tangent equation is: \[ 4x + y = 28 \]